偏微分方程团队现有博士11人,硕士1人,其中教授2人,副教授4人,讲师5人。团队主要关注流体力学中的偏微分方程、反应扩散方程、非线性色散波方程散射理论中的应用、数学物理与可积系统等。
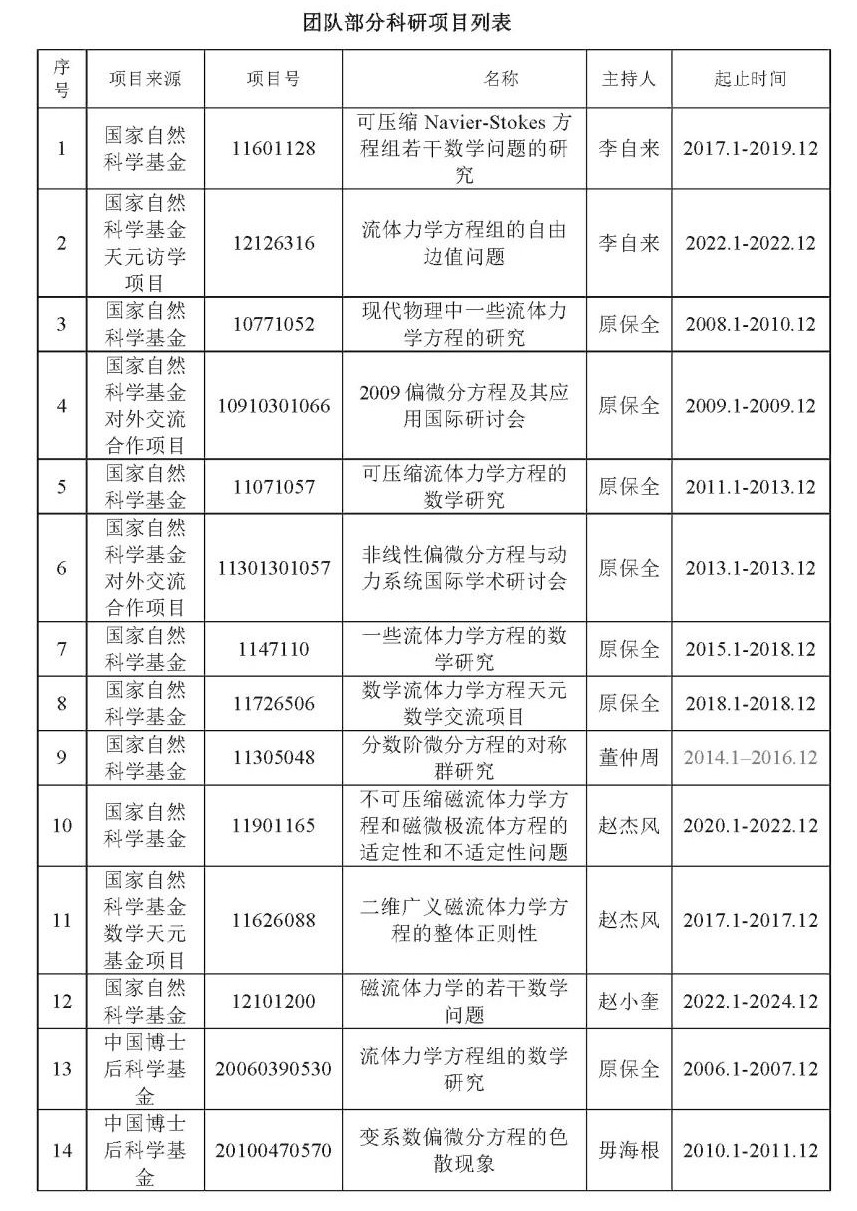
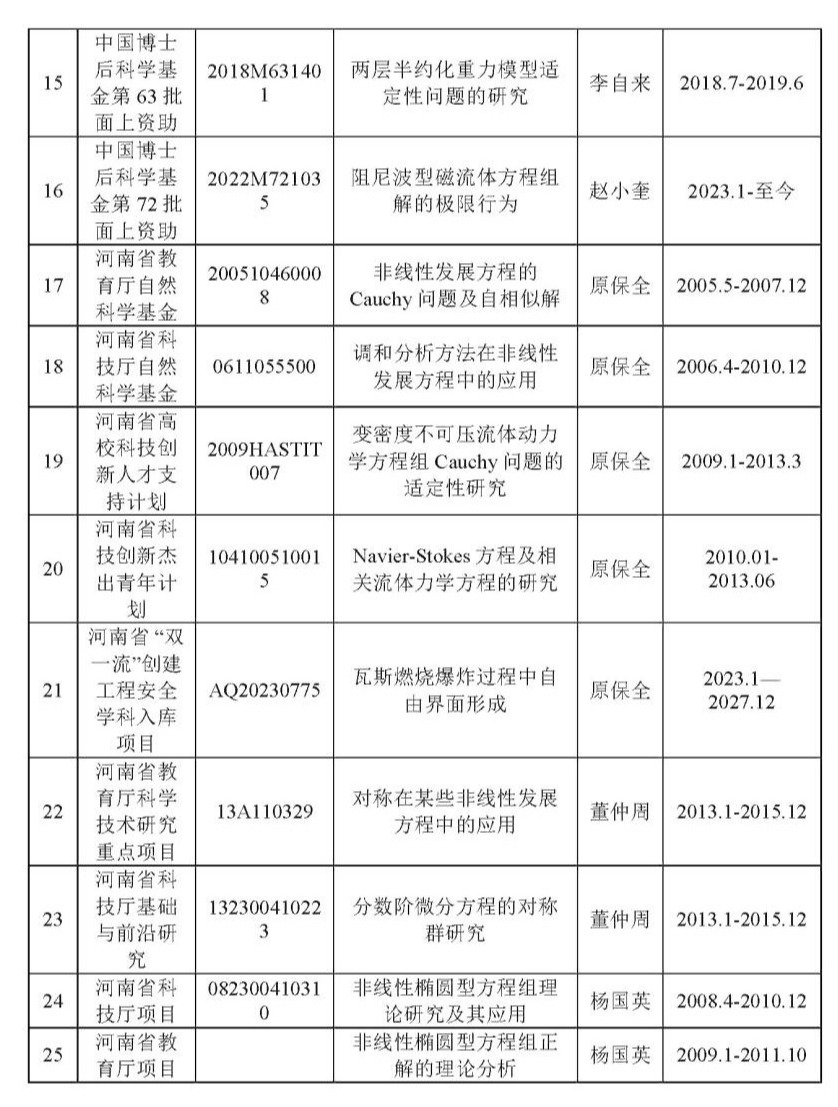
偏微分方程是数学的核心研究领域之一,经过数百年的发展,偏微分方程被广泛应用于物理学、工程学、计算机图形学、金融学、生物学等领域。本团队致力于研究流体力学方程组的适定性,稳定性和粘性消失极限;反应扩散方程组的理论,现代调和分析和黎曼几何方法在非线性色散波方程散射理论中的应用、非线性数学物理与可积系统等问题。成果发表在J. Math. Pures Appl., SIAM J. Math. Anal., Int. Math. Res. Not. IMRN, Calc. Var. Partial Differential Equations, J. Differential Equations, J. Math. Fluid Mech., Science China-Mthematics, J. Evol. Equ., Commun. Math. Sci.等期刊上,主持国家自然科学基金12项,博士后基金4项,省部级项目9项。



代表性论文列表
[1]Zilai Li, Hao Liu, Yulin Ye, Global classical solutions to the viscous two-phase flow model with Navier-type slip boundary condition in 2D bounded domains, J. Math. Fluid Mech., 24 (2022), Paper No. 85, 34 pp.
[2]Kunquan Li, Zilai Li, Yaobin Ou, Global axisymmetric classical solutions of full compressible magnetohydrodynamic equations with vacuum free boundary and large initial data. Science China-Mthematics, 65(2022), 471-500.
[3]Lei Yao, Zilai Li, Wenjun Wang, Existence of spherically symmetric solutions for a reduced gravity two-and-a-half layer system. J. Differential Equations 261 (2016), 1637-1668.
[4]Lei Li, Wantong Li, Mingxin Wang, Dynamics for nonlocal diffusion problems with a free boundary, J. Differential Equations, 330 (2022), 110–149.
[5]Weijie Sheng, Mingxin Wang, Zhicheng Wang, Front propagation and blocking of time periodic bistable reaction-diffusion equations in cylindrical domains. Calc. Var. Partial Differential Equations 62 (2023), Paper No.173, 65 pp.
[6]Yihong Du, Mingxin Wang, Semi-wave and spreading speed for the diffusive competition model with a free boundary. J. Math. Pures Appl. 107 (2017), 253–287.
[7]Chongsheng Cao, Jiahong Wu, Baoquan Yuan, The 2D incompressible magnetohydrodynamics equations with only magnetic diffusion, SIAM J. Math. Anal. ,46 (2014) , 588-602.
[8]Baoquan Yuan,Jia Yuan, Global well-posedness of incompressible flow in porous media with critical diffusion in Besov spaces, J. Differential Equations, 246 (2009) 4405-4422.
[9]Haigen Wu ,Yue Hu, On Variational iteration method for fractional calculus, Themal Science, 121 (2017), 1707-1721.
[10] Changxing Miao, Haigen Wu, Junyong Zhang, Scattering theory below energy for the cubic fourth-order Schrodinger equation, Math. Nachr., , 288 (2015), 798–823.
[11] Jiahong Wu, Jiefeng Zhao, Mild ill-posedness in L∞ for 2D resistive MHD equations near a background magnetic field, Int. Math. Res. Not. IMRN, (2023) 4839–4868.
[12] Jiahong Wu, Jiefeng Zhao, Global regularity for the generalized incompressible Oldroyd-B model with only stress tensor dissipation in critical Besov spaces, J. Differential Equations, 316 (2022), 641–686.
[13] Ying Sun, Jianwen Zhang, Xiaokui Zhao, Nonlinearly exponential stability for the compressible Navier-Stokes equations with temperature-dependent transport coefficients, J. Differential Equations, 286 (2021), 676-709.
[14] Yanghai Yu, Hui Wang, Jinlu Li, Xiaolei Yang, Global solutions to the 3D compressible Navier–Stokes equations with a class of special initial data, Journal of Mathematical Physics, 64 (2023),Paper No.021502, 16 pp.


(图为2009年举办偏微分方程及其应用国际学术研讨会)
(图为2023年举办偏调和分析及在 PDEs、数论与几何测度论中的应用研讨会)
(图为2013年举办非线性偏微分方程与动力系统国际学术研讨会)
(图为2018年举办数学流体力学方程国际研讨会)
(图为2023年团队成员参加偏微分方程建模与理论学术讨论会合影)
(图为2023年团队成员参加第十届偏微分方程青年学术论坛合影)
